The Exponential Growth Curve

Snaphanen has posted some interesting figures today on the growth of the Muslim population in Sweden:

Swedish Muslims

1970		1,000
1980		30,000
1985		50,000
1988		100,000
1990		120,000
1992		140,000
1994		160,000
1996		200,000
1998		250,000
2000		325,000
2005		375,000
2006		400,000

Table 1. Estimated Muslim population (1970-2006) [i Sverige]
Source: Sander and Larsson

When I looked at those figures, my natural inclination was to plot them in a graph. Here’s the result:


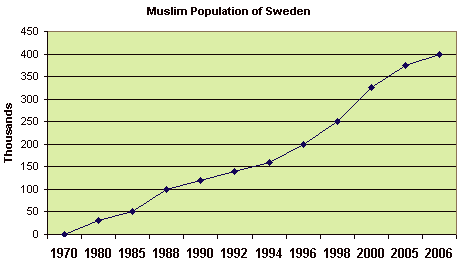
At first glance this graph shows a steady growth, which may or may not be alarming to the average Swede. However, that growth appears to be a simple linear trend, with the figures rising in more or less a straight line.

But this graph is deceptive. The number of years between successive population figures is much greater in the earlier part of the table than it is for the more recent figures. To make it easier to see the trend in the graph, I added the missing years and inserted the estimated population as a standard proportional interpolation. That makes the graph look quite different:
- - - - - - - - -

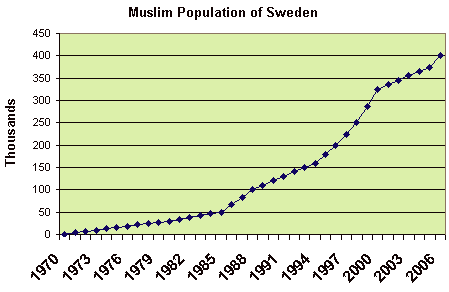
The revised graph does not show a linear trend. It’s too early to tell for sure, but that looks like an exponential curve to me. Here’s an example of an exponential growth curve:


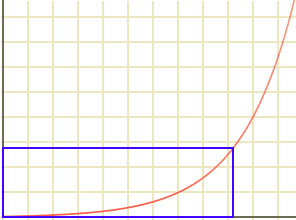
The rectangle marked in blue represents the period shown in the second population graph. As you can see, in the coming years the curve rapidly steepens.

An exponential increase means that the population doubles within a certain fixed period. Suppose you started at 1980 with 30,000 (the same figure as in the actual statistics) and doubled it every 6.7 years. The resulting population table would look like this:

1980		30,000
1987		60,000
1993		120,000
2000		240,000
2007		480,000
2014		960,000
2020		1,920,000
2027		3,840,000
2034		7,680,000
2040		15,360,000
2047		30,720,000

These figures come close to the actual numbers listed since 1980. If the trend continues, since the population of Sweden in 2007 is estimated to be between 9 and 10 million people, within about thirty-five years there will be more Muslims than “persons of Swedish origin” in the country. And this is assuming that there is no further emigration from Sweden of the native population.

*   *   *   *   *   *   *   *   *   *   *   *   *   *   *
Obviously, the future demographics will not really look like this. Even if the rate of increase actually is linear, and not exponential, something will change — either Sweden will resist further Islamization, and the Muslim population will stabilize at a lower level, or Sweden will succumb. In the latter case the numbers will stabilize at a population which can be supported by a slave-based feudal economy within the land area available.

The population fluctuations for any species in a real ecosystem exhibit chaotic characteristics. In the typical case, there is an initial slow start, with an exponential growth curve causing an explosive increase after a certain amount of time. Then the limiting factors kick in — exhaustion of the food supply, an increase in natural predators, disease through overcrowding, and so on. After that, the population crashes, and the process begins all over again with the survivors forming the nucleus of a new population explosion. The resulting cycles, being chaotic in nature, are generally not predictable.

Other limitations on exponential growth may appear as a result of the way the data are defined. For example, consider the increase in illegitimate births in the United States. Over the last forty years or so the increase of illegitimacy has exhibited the characteristics of exponential growth. In recent years, however, that increase has slowed.

Is that because young Americans are becoming less promiscuous and more responsible? No; it’s because the proportion of illegitimate births, at least in certain subgroups, is approaching the natural limit of 100%.

Both examples illustrate the fact that an exponential growth trend cannot continue past the carrying capacity of the environment in which the growth takes place.

In Sweden’s case, the limiting factor which will define the county’s carrying capacity for Muslim immigration has not yet become apparent.